Compound Interest Calculator - Future Value Of An Investment

This is a very useful compound interest calculator. Here you can calculate the future value of an initial deposit/investment after a specified period (in years) rate of interest.

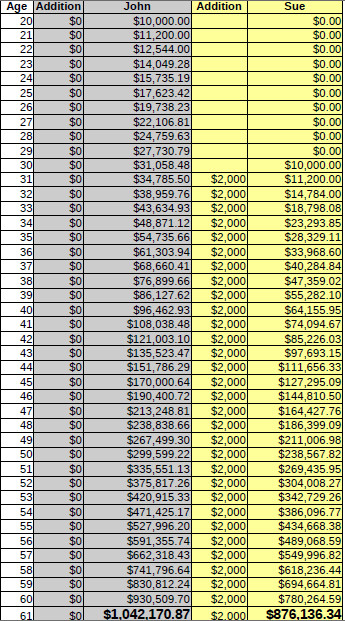
This is a quick guide of how to use the compound interest calculator:
1. "Enter an Initial amount" - this is the starting amount for the calculation
2. "Annual addition" - if you plan adding to your initial amount every year, you can enter it here.
3. "Interest rate / ROI in %" - This is the field where you have to enter the interest rate percentage. Type it just as a number, without the "%" sign after it.
4. "Number of years:" For how many years do you want to calculate the compounding. This is the period of the investment/deposit in years.
5. "Reinvested profit %:" - Every year you earn interest on your money. Do you plan to withdraw it and what part of it? If you don't want to withdraw any percentage of your earned interes yearly, leave this "100", which means that you reinvest all (100%) of the earned interest.

Using this calculator you can calculate things like: how long would it take to make a million of dollars investing 5 dollars a day. In fact this is completely possible, you can see some proofs here: https://businessideaslab.com/earn-a-million-investing-5-dollars-daily/

Compounding Interest Calculator

If You Want To Get Rich Compounding, Start Young

As we see on this blog, compounding can make you rich. It's slow, but it CAN make you rich. According to me, It's actually not the best way to make it, because you will end up as a rich pensioneer, but it's a real way to do it. So, if you have chosen this way to wealth, here is one really important thing you should know about. This is one of the most important factors in the wealth equation, and it's to start early in life.

Once Warren Buffett said, that the best time to start investing is 20 years ago, and the second best time is ... now. The earlier you start doing it, the most advantage you take of the power of compounding. To give you a proof, lets examine the following example in the table below.

John started investing when he was 20 years old. The only thing he did is to put $10 000 into a mutual fund. Then he just forgot about his investment for the next 41 years.

Sue decided to start investing like John, but this happened when she was 30. In order to catch him, she decided to put $10 000 dollars in the same mutual fund and also to add $2 000 every year from then on.

The mutual fund yielded 12% on average, this is the average return of the US stock market.

Years passed and John and Sue got 61 .  The $10 000 investment of John has compounded to more than a million dollars and he became a millionaire. Sue started later, but she did her best to catch up with John, adding $2 000 every year to her same investment of $10 000. But she never reached John. He had only $876 000 .

This is why it's totally important to start as early as possible.

If you like this post, please, share it with friends.

Compound Interest Formula

Here we will go through some compound interest formulas , used for the calculations in compounding. The very basic formulas associated with compound interest are these for the future value of money and the present value of money.

Basic Compound Interest Formulas

The future value compounding formula - shows what an investment would be after a certain period (in the future) compounded at a specific rate:

FV = PV(1+i)ⁿ
FV - future value
PV - present value
i - interest rate

Exampe: The future value of $100 dollas at 10% annual interest rate after 5 years would be:
FV=100(1+0.10)⁵ = 100*1.61051 = $161,05

You can easily calculate the future value of and investment with our compound interest calculator

The present value compounding formula - - shows the present value of a future amount of money.

PV = FV/(1+i)ⁿ

where
FV - future value
PV - present value
i - interest rate

Now let's see a simple example:
Example: John wants to pay me $1000 after 3 years, if I gave him $800 today. Would it be beneficial for me, if I can receive 7% interest on my money in the bank?

To solve this, we should see what would be the present value of the future payment of $1000 dollars, and see if it is greater than $800. Is so, we will make a profit lending to John.

PV = 1000/(1+0.07)³ = 1000 / 1.225043 = 816.2978768909

So the future value is greater than the amount I would give to John with  $16.28 . This will be my profit if I lens him.

You can read a lot on the matter here.

Compound Interest Definition In Simple Words

What Is Compound Interest?

The following is a simple explanation of compound interest and the so called compounding. So lets say that we have a thousand dollars ($1000), which we would like to invest in a bank deposit for 5 years. The bank gives us 10% interest yearly on our money, because they use it for their purposes, as long as we keep the money with them. So this is what happens with the invested money year after year:

 Year 1 - After the first year, we should receive 10% on our initial deposit of $1000, which is $100 dollars in interest earning. Now we have $1000 deposit + $100 interest, or our initial deposit has grown to $1100, which is the starting amount for the second year.

 Year 2 - After the second year our 10% interest equalls 10% from $1100 or $110. We get $10 more than the interest from the first year, because the interest from the first year itself has earned interest. That's how with our initial deposit of $1000, after the second year we earn $110 or more than 10%

This is the sweetest thing about compound interest: the earned interest earns interest itself!

After years 3,4,5 our interest earnings from the initial depost of $1000 will be:
Y3 - $133
Y4 - $146
Y5 - $161

This is what happens with the deposit year after year:

Albert Einstein called compounding interest "The greatest mathematical discovery of all time!

Amazing! We earn more and more from our initial deposit of $1000 every next year! This is called compounding, or compound interest. After the 10th year we will earn in interests - $1594! Thats great! And this is only from a single investment of $1000. Yes, it is great!Albert Einstein called compounding interest "The greatest mathematical discovery of all time!" an it is!

Do you know what your earnings would be from this initial deposit of $1000 after the 30th year! Believe me, or not, your earnings in interest will be the amazing 16 449 US dollars!

Check it out with our compounding interest calculator, where you can define different periods, initial deposits and compound interest rates!

This is how some of the richest people in the World has made their fortune - Warren Buffet for example!

Compounding Interest Rate Chart

Here is an example chart of the compounding interest rate, in which a $100 deposit is invested for 40 years, earning 10% interest rate yearly. As you can see there is an exponential increase of the value of the deposit over the years:

Only 10% compound interest rate is needed to make $4525,93 from an initial investment of only $100 and no work at all :) ! This is an example of how money can work for you! On the following picture, you can see the detailed increase of the investment calculated with the compounding interest rate calculator here in this site. This is a nice chart where you can see the growth of your money during the years.